Did You Solve It? Oxford University Admissions Questions
As we are approaching a looming deadline for Oxbridge applications on 15 October, here’s something to flex your brain in preparation for the next step – the interview (for those who get the invitation, of course!).
During the induction day last Monday, we heard first-hand from one of our former Cherwellians (Haiqi) about his experience going through Oxford University interviews at three Oxford Colleges. Haiqi ended up reading Maths at Corpus Christi College, University of Oxford and was sharing some memorable episodes with the new cohort of Cherwellians as to what it’s like to be interviewed by Oxford professors! It is indeed a very varied and to an extent unpredictable experience, you can be put off-guard by being asked a seemingly ‘simple’ question followed by a more complex one down the line… However, everything very much depends on the interviewer and their approach. A key take-away: if you do well on your aptitude test, you are likely to do well in your interviews! So do your homework and prepare well for the aptitude test required for your course, practise as many questions and read as much material as possible, start your preparation early, do a set of mock interviews and practise answering questions verbally as well as on paper.
There may well be more than one right answer to a question (or no right answer at all!), in which case, the interviewers will be more interested in exploring the applicant’s thought process and how they approach a question or a task in hand.
Epistemic logic puzzles have been set in recent years during interviews for joint philosophy degrees. Joel David Hamkins, who is Oxford’s Professor of Logic and the Sir Peter Strawson Fellow in Philosophy at University College, Oxford, shared a few conundrums with The Guardian recently. And we are wondering if you could solve them right off! Ready to tease your brain?
The answers to the logical conundrums will be posted right after the puzzle. Please no peeping before you solve it!
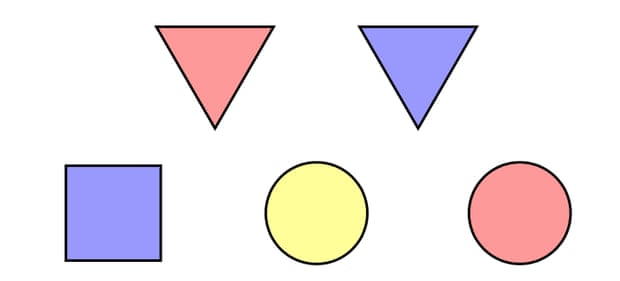
Can You Guess The Tile?
The perfectly logical philosophy friends Sheila and Colin know there’s a surprise hidden under one of these coloured tiles. Each friend is privately told a piece of information about where the surprise is: Sheila knows only the shape of the tile; Colin knows only the colour of the tile. It is commonly known by all that this and no other information is given.
Host: Do either of you know where the surprise is?
[Awkward long silence]
Host: Do you know now?
[More awkward silence]
Sheila, Colin: (simultaneously): Now I know where it is!
So, where is the surprise?
The train of thought:
The only way that Sheila could know the correct tile before the dialogue is if she had been told ‘square’, and the only way that Colin could know is if he had been told ‘yellow’. Since they are silent when the host asks the first question we assume that they don’t know the correct tile, and thus we can eliminate the square and the yellow tiles.
If Sheila had been told ‘circle’ she would now know the correct tile, because there is only one circle left. If Colin had been told ‘blue’ he would also know the correct tile. However since both of them remain silent when the host asks the second question, we can assume that they don’t know the correct tile, and thus we deduce that the tile must be red and a triangle.
Solution: The surprise is under the red triangle.
That was only a starter? Ready for the main course? 🙂 Here’s another puzzle from Mr. Hamkins:
Alice’s Boxes
Alice has invited her friends Caroline and Susan to her home, and she has placed several boxes on the table before them. They are all perfectly logical.
- small red box
- medium red box
- large black box
- small blue box
- large blue box
Alice tells her friends that she has placed a small gift into one of the boxes, and she has privately told Caroline the colour of the box and Susan the size of the box, and they both know this. The following conversation ensues:
Caroline: I don’t know which box contains the gift, and I also know that Susan doesn’t know.
Susan: I already knew before you spoke that you didn’t know which box contains the gift.
Caroline: Ah, now that you say that, it suddenly occurs to me which box must contain the gift.
Question. Which box contains the gift?
The train of thought:
If Caroline doesn’t know the box, she cannot have been told ‘black’. And if she knows Susan doesn’t know, Caroline can’t have been told ‘red’, since if she had been told ‘red’ then there is a chance Susan had been told ‘medium’, in which case Caroline would not be able to say that she knows Susan doesn’t know. So we deduce Caroline was told ‘blue’.
Now if, before Caroline spoke, Susan knew that Caroline didn’t know the correct box, we can eliminate the possibility that Susan was told ‘large’. Since if she had been told ‘large’ she would not know for certain that Caroline didn’t know, since she cannot rule out the possibility that the large black box has the gift, in which case Caroline would know. Since the box is blue and not large, the only remaining option is small and blue.
Solution: small blue box
Do you want to solve the epistemic logic puzzles as easy, as shelling peas? Or be fully prepared before the interview? Cherwell College Oxford provides Oxbridge preparation courses. To find out more or book a personal consultation, please visit: Oxbridge Preparation